Delta, Theta, Gamma – no, we are not revisiting your traumatic memories of high school math class. These are not the equations that haunted your dreams or left you scrambling for the calculator.
Instead, we are diving into a world where these mathematical-sounding terms, called option Greeks, take on a whole new meaning – a meaning that can transform the way you approach options trading.
Let’s delve deeper into how these options Greeks help us gauge the risks and rewards of options trading.
You may also like: Delta hedging – A comprehensive guide on mechanics and implications
What are options Greeks?
Options trading is one of the most challenging forms of trading due to its intricate nature. It encompasses a lot of concepts that options traders must be well-versed in to effectively navigate the market. One such concept is options Greeks.
Options Greeks refer to a set of risk measures named after Greek letters.
They gauge an option’s sensitivity to various factors, such as:
- Time-value decay,
- Interest rates
- Changes in implied volatility, and
- Movements in the price of its underlying security.
These measures play a pivotal role in determining an option’s price and are extensively employed by successful traders when buying or selling different options.
The primary types of option Greeks are:
- Delta,
- Vega
- Rho
- Gamma and
- Theta
If you understand the intricacies of option chain Greek, you can make informed decisions and effectively manage your options trading strategies.

Delta: How stock prices impact options’ price
The Delta option Greek estimates the impact of changes in the underlying asset’s price. In simple terms, it shows how the price of a call-and-pull option shifts with the changes in the underlying asset’s price.
For example, an option Delta of 0.20 means that the option’s price will theoretically change by ₹0.20 for every ₹1 change in the underlying stock’s price.
If options have a higher Delta, then it means that they are more volatile to shifts in the underlying asset’s price.
There is a specific range for Delta, i.e., -1 to 1. Call and put options both have different Delta ranges. For call options, the range is from 0 to 1 and for put options, the range is from -1 to 0.
The formula to compute Delta is:
Delta: ∂V/AS
Here,
∂ = the first derivative
S = the underlying asset’s price
V = the option’s price
Options closer to In-the-Money (ITM) will have a Delta closer to 1 in the case of call options and -1 in the case of put options.
If you understand the Delta, you can easily hedge your position and make informed trading decisions.
Also Read: Futures vs. Options: Differences every investor must know!
Gamma: The change in Delta
Gamma is a measure that reveals how an option’s Delta will respond when there is a 1-point change in the underlying asset’s price. In simpler terms, Gamma option Greek demonstrates the sensitivity of an option’s Delta to market price fluctuations.
Understanding Gamma is crucial as it provides insights into the pace at which an option’s Delta alters in response to changes in the market price of the underlying asset. However, it is not typically required for calculating most option trading strategies.
Options that are at-the-money (ATM) tend to have higher Gamma values, indicating a greater sensitivity to market price changes.
Theta: How time impacts options’ price
Theta is a measure that quantifies the change in an option’s price relative to the change in its time to maturity. Options are often referred to as depreciating assets since their premiums tend to lose value as the expiration date approaches.
Theta, also known as time decay, has a significant role when it comes to options trading. If an option’s time to maturity decreases by any amount, the option’s price will change by an amount equal to its Theta.
For instance, if a trader has purchased a call option with a Theta of -0.60, the price of the call option would decrease by 60 paisa each day, assuming other factors remain constant.
Theta values intensify as the time to expiration draws near for a given option.
Also Read: What is an F&O ban? Know its impact on futures and options trades
Vega: How implied volatility impacts options’ price
Vega is one of the five primary options Greek that traders use. Specifically, Vega quantifies the impact of the implied volatility of the underlying stock on an option’s price.
When an option has higher implied volatility, its price benefits as it increases the likelihood that the stock’s price will eventually land in the money (ITM).
Vega option Greek provides an approximation of how much an option’s price will increase or decrease when there is a corresponding increase or decrease in the level of implied volatility.
For instance, let’s assume that an option has a Vega of 0.3. If the underlying asset’s implied volatility rises by 2%, then the option’s price would increase by ₹0.60. It is vital to note that a rise in Vega will increase both call and put options prices.
Rho: How interest rates impact options’ price
Rho is another trading option for Greeks but is not often used. Basically, it gauges how the option’s price changes with a shift in the interest rate. It provides insights into how the price of an option should rise or fall when the risk-free interest rate, such as treasury bills, increases or decreases.
When interest rates increase, the call option’s value increases and the put option’s value usually decreases. Due to these characteristics, a call option has a positive Rho, while a put option has a negative Rho.
The image below depicts how options Greeks look on an option chain.
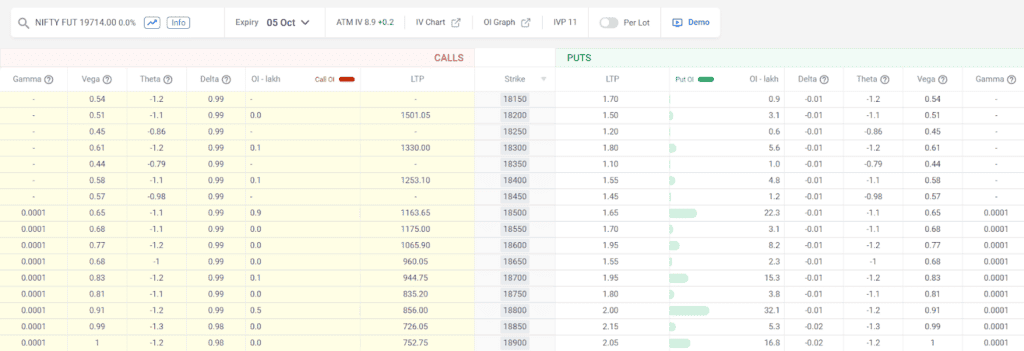
Source: Sensibull
Here, as we can see, call options have a Delta in the range of 0 to 1, and put options have a Delta in the range of -1 to 0.
Here, a Delta of 0.99 means that the option’s price will change by ₹0.99 for every ₹1 change in the underlying stock’s price.
Further, for a call option with a Theta of -1.20, the option’s price would decrease by ₹1.2 each day, assuming other factors remain constant.
Bottomline
Option Greeks like Delta, Gamma, Theta, Vega, and Rho show us how changes in underlying stock price, implied volatility, time-value decay, and interest rates affect the options pricing. Using these Greeks, traders can make successful trading strategies and maximise their returns.
In addition to the Greeks, experts also recommend leveraging other analysis tools, such as charts and technical indicators, to make more informed decisions.


Leave a Comment